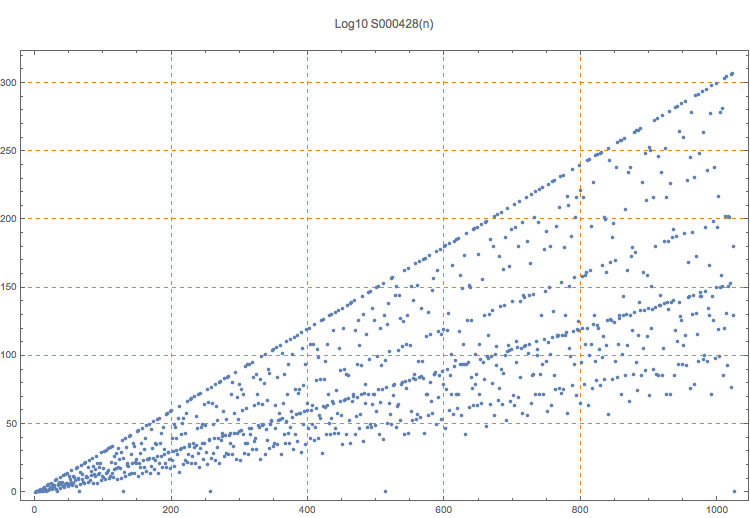
The Bombieri norm of Phi(n,x), the n-th cyclotomic polynomial.
1, 2, 2, 4, 2, 16, 4, 64, 2, 22, 16, 1024, 8, 4096, 64, 200, 2, 65536, 22, 262144, 128, 2380, 1024, 4194304, 72, 215766, 4096, 48622, 2048, 268435456, 200, 1073741824, 2, 765248, 65536, 14136356, 926, 68719476736, 262144, 10228426, 16512, 1099511627776, 2380
0
Note that the norm is 2 for all n = 2^k with k >= 0. When n is prime then the term is 2^(n-1).
T. D. Noe, Plot of 1025 terms
T. D. Noe, Table of 1025 terms
Eric W. Weinstein, MathWorld: Bombieri Norm
(Mma) Table[c = CoefficientList[Cyclotomic[n, x], x]; deg = Length[c] - 1; Binomial[deg, Range[0, deg]].Abs[c], {n, 0, 50}]
Cf. A051664, A160338, S000426, S000427.
nonn,nice
T. D. Noe, Dec 19 2014